Radian

The radian, until 1995, used to be the standard unit of angular measurement. Nevertheless, this unit of measurement is often used in many fields of mathematics.  Even though its unit symbol may be "radian" or "rad," it is usually omitted. Therefore, radian is called a dimensionless quantity.  

Mathematically speaking, a radian is the value of the division of a certain arc length with the radius of the arc. One radian is equal to 57.3 degrees, therefore it may be concluded that one full circle has a radian equal to 2π. Considering that a circle has 360 degrees, therefore, one radian will be equal 180/π degrees.

When you need to convert radians into degrees, you must multiply the value you have by 180 and the divide the result by pi. If you have your values and degrees, and wants to convert to radians, take your values and multiply by pi, then divide by 180. 

The radian measure was first used in opposed to the degree of an angle by Roger Cotes in 1714. However, the term radian was only used in print form on 5^th June of 1873, in examination questions set by James Thomson, at Queen's College, Belfast.  

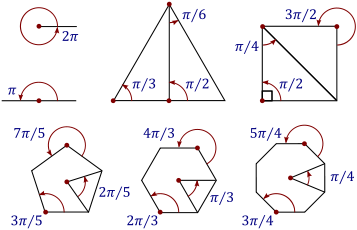It is important to understand the concepts of radian measures because, in calculus, angles are universally measured in this unit. The reason for this is because it can lead to a more elegant formulation of a number of important results than degrees of angles would, since radian is a pure measure based on the radius of the circle. When the results in analysis involves trigonometric functions for example, radians are used in order to have the results expressed in a more elegant and simple way. To conclude the thought: "Degrees are more practical, but radians are more elegant and mathematically easier."